I am interested in doing some high-magnification macro photography, which is something I've never done before. I've never worked over 2:1, and I plan to explore the region between 3:1 and 10:1. To that end, I've purchased a Zeiss S Planar 50mm f/1.6 lens. That lens was designed to be used for semiconductor photolithography in the 1980s. In the intended application, It operated with a mask set that was ten times the size of the image projected on the wafer, and was thus intended to be a 1:10 lens. In that application, it was capable of resolving about 1500 line pairs per millimeter. I figure if I use it backwards, I can get 10:1 magnification that will resolve about 150 lp/mm.
The lens has a significant limitation. It was designed to be used with monochromatic light of 436 nm wavelength. No color images with this lens, unless you love extreme amounts of longitudinal chromatic aberration.
Good optics at these magnifications are usually diffraction limited. That means that the Q calculation that I talked about in this blog many years ago is a good way to find out when reducing the pixel pitch of the sensor will no longer yield better images.
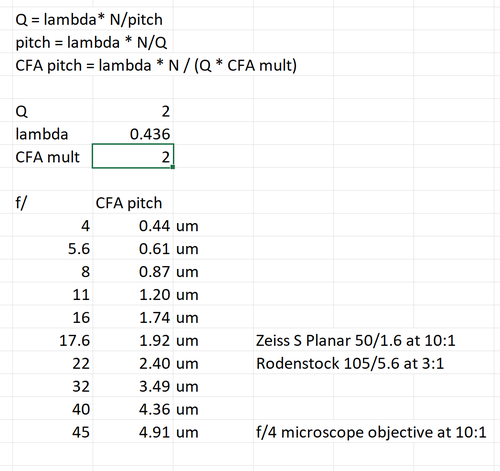
I created this spreadsheet:
At the top are the formulae I used. Q is the metric for determining whether the pitch of the sensor and the resolution of the lens are "balanced", which occurs at Q = 2 for a monochromatic sensor and is defined as the coarsest pitch which will produce no aliasing with a diffraction-limited set of optics. When used with a Bayer color filter array (CFA) sensor, a fudge factor needs to be introduced to account for the coarser sampling in each color plane. My usual compromise fudge factor for full color images is 1.7, but in this case I wanted to account for monochromatic blue light so I used a factor of 2.
The lefthand column on the lower part of the spreadsheet is the effective f-stop, which at these magnifications is the nominal f-stop times one plus the magnification. The righthand column on the lower part of the spreadsheet is the coarsest pitch that won't show aliasing. With my Zeiss S Planar at 10:1, that's 1.74 micrometers (um), which is about half of the pitch of the GFX 100S I plan to use with this lens. So if my setup works and there's no vibration -- an issue at my new second-floor location -- the camera will be the limiting factor, not the lens.
I've also included a row for my Rodenstock 105mm f/5.6 HR Digaron at 3:1. If a fudge factor of 1.7 instead of 2 is used, the camera is still the limiting factor. If we use 550 nm light and a fudge factor of 1.7, the critical sensor pitch is 2.74 um, still finer than the GFX 100S sensor pitch.
Although I'm not using microscope objectives at present -- they typically don't cover the GFX 100S sensor, I included an f/4 objective used at 10:1 for comparison. Used with such a lens, the GFX 100S samples a bit more finely than it needs to.
In the real world, there are lots of possible blurs sources other than diffraction at high magnifications: misfocusing, depth of field, vibration. So the above is probably somewhat overconservative. On the other hand, the Q = 2 calculation assumes optimal reconstruction, so it is slightly underconservative.